Rational root theorem
In algebra, the rational root theorem (or rational root test, rational zero theorem, rational zero test or p/q theorem) states a constraint on rational solutions of a polynomial equation aₙxⁿ+aₙ₋₁xⁿ⁻¹+⋯+a₀=0 with integer coefficients aᵢ∈ℤ and a₀,aₙ≠0. Solutions of the equation are also called roots or zeroes of the polynomial on the left side. The theorem states that each rational solution x = p/q, written in lowest t…
Full Answer
How to find all possible rational roots?
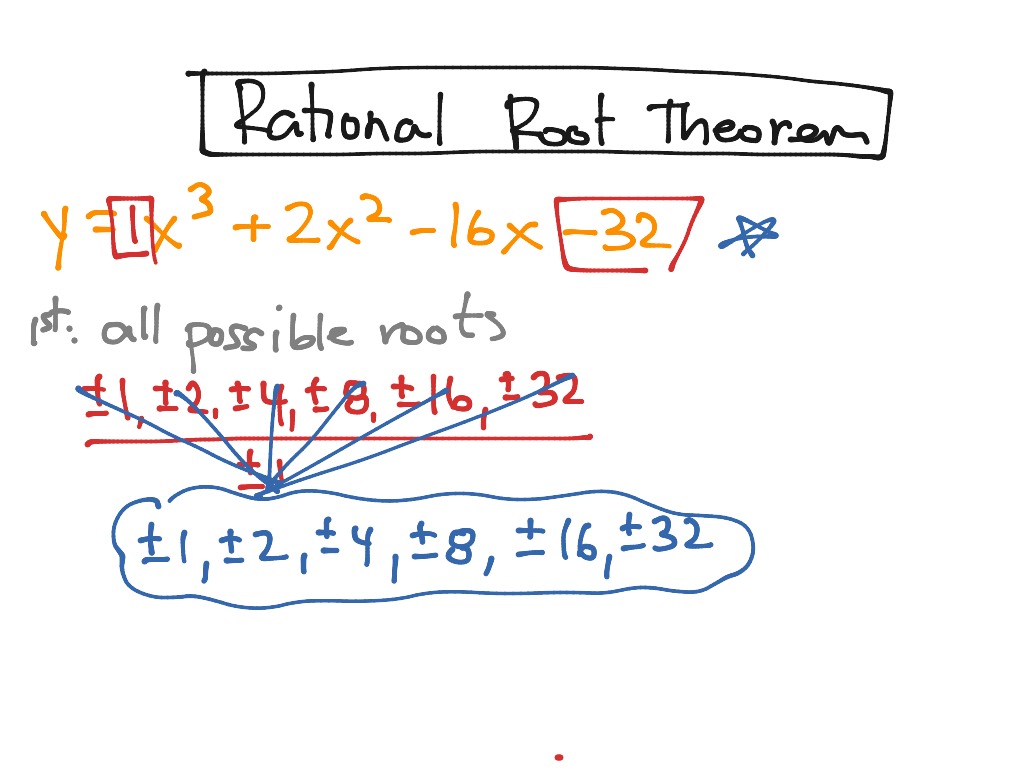
a) To find the possible rational roots, use the theorem: ± the factors of the constant-coefficient, 42, divided by the factors of the x 3-coefficient, 2. b) For each possible rational root, replace x with the value and evaluate the function. c) The confirmed roots are the ones that made the function equal to zero.
What does rational root mean?
What does it mean to be free in our world ... 26 million of them refugees outside their own borders. Ultimately, the root causes of this crisis are a tangle of political, economic and ecological factors that push people to escape, coupled with an extreme ...
What is a 'unique real root'?
The roots that are found when the graph meets with the x- axis are called real roots; you can see them and deal with them as real numbers in the real world. Also, because they cross the x- axis, some roots may be negative roots (which means they intersect the negative x- axis), and some may be positive roots (which intersect the positive x- axis).
What are the real roots of?
The term real root means that this solution is a number that can be whole, positive, negative, rational, or irrational. While numbers like pi and the square root of two are irrational numbers, rational numbers are zero, whole numbers, fractions and decimals. However, the solution to an equation can be real roots, complex roots or imaginary roots.
What is a rational root in math?
rational root theorem, also called rational root test, in algebra, theorem that for a polynomial equation in one variable with integer coefficients to have a solution (root) that is a rational number, the leading coefficient (the coefficient of the highest power) must be divisible by the denominator of the fraction and ...
What are rational roots example?
Rational Root Theorem Examples Example 1: Find the possible rational zeros of the cubic function f(x) = 3x³ - 5x² + 4x + 2. Solution: The constant term is 2 and its factors are ± 1 and ± 2. ... Then by the rational zero theorem, the possible rational roots of f(x) are all possible values of p/q.
How do you know if a root is rational?
0:076:51Rational Roots Test / Theorem - YouTubeYouTubeStart of suggested clipEnd of suggested clipBut it says if there are rational roots it says to get them you basically look at factors of theMoreBut it says if there are rational roots it says to get them you basically look at factors of the constant. You look at factors of the leading coefficient. You look at all possibilities.
What is a real rational root?
When a zero is a real (that is, not complex) number, it is also an x-intercept of the graph of the polynomial function. ... The Rational Roots (or Rational Zeroes) Test is a handy way of obtaining a list of useful first guesses when you are trying to find the zeroes (roots) of a polynomial.
What are irrational roots?
The answer is yes! The irrational root theorem can be used to find additional roots for a polynomial. ... The irrational root theorem states that if the irrational sum of a + √b is the root of a polynomial with rational coefficients, then a - √b, which is also an irrational number, is also a root of that polynomial.09-Feb-2022
How do you find all rational solutions?
0:2612:17Finding All Zeros of a Polynomial Function Using The Rational Zero ...YouTubeStart of suggested clipEnd of suggested clip1 is just plus or minus 1 and any number divided by 1 is itself. So therefore the possible rationalMore1 is just plus or minus 1 and any number divided by 1 is itself. So therefore the possible rational zeros are 1 2 3 & 6. So if we set this function equal to 0.
How do you illustrate rational root theorem?
1:476:10Rational Root Theorem - YouTubeYouTubeStart of suggested clipEnd of suggested clipThis is going to be 2 over 1 which is 2 so these all could be positive or negative 3 4 6:12 or itMoreThis is going to be 2 over 1 which is 2 so these all could be positive or negative 3 4 6:12 or it could be 1/2.
How does rational root theorem helps in solving equations?
The rational roots theorem is a very useful theorem. It tells you that given a polynomial function with integer or whole number coefficients, a list of possible solutions can be found by listing the factors of the constant, or last term, over the factors of the coefficient of the leading term.20-Oct-2021
Do rational roots come in pairs?
The short answer is NO.02-Oct-2010
How do you do Ps and Qs in math?
0:234:33How to use p over q to find the possible and all zeros - YouTubeYouTubeStart of suggested clipEnd of suggested clipThat's can equal plus or minus three. And over plus or minus one all over Q which is going to beMoreThat's can equal plus or minus three. And over plus or minus one all over Q which is going to be plus or minus four comma plus or minus two comma plus or minus one.
Is 32 an irrational number?
32 is not an irrational number because it can be expressed as the quotient of two integers: 32 ÷ 1.
How do you find imaginary roots?
Imaginary roots appear in a quadratic equation when the discriminant of the quadratic equation — the part under the square root sign (b2 – 4ac) — is negative. If this value is negative, you can't actually take the square root, and the answers are not real.21-Dec-2021
What is rational root?
As the name suggests, a rational root is the combination of a rational number with a root. The rational root theorem, which is also called the rational zero theorem, says that any rational roots of the polynomial must be one of the following: Don’t forget your handy quick reference guide for factors.
How to find rational roots?
a) To find the possible rational roots, use the theorem: ± the factors of the constant-coefficient 12 divided by the factors of the x 4 -coefficient 1.
When to use rational root theorem?
Only use the rational root theorem when the coefficients are small, and as a last resort or when you’re told to. It is less efficient than some other steps you could take first.
What does putting x= -2, 1 3, 3 give?
If f (x)=0, then the value is a rational root, so you can see that putting x= {-2, 1 ⁄ 3, 3} gives 0.
What is root in math?
A root is a value for x that makes the function equal to zero. It is also called a solution.
Why is root x=-1 repeated?
In the case above, the root x=-1 is repeated because it is also a local maximum.
What is confirmed root?
c) The confirmed roots are the ones that made the function equal to zero.
Cubic equation
with integer coefficients has three solutions in the complex plane. If the rational root test finds no rational solutions, then the only way to express the solutions algebraically uses cube roots.
Proof using Gauss' lemma
Should there be a nontrivial factor dividing all the coefficients of the polynomial, then one can divide by the greatest common divisor of the coefficients so as to obtain a primitive polynomial in the sense of Gauss's lemma; this does not alter the set of rational roots and only strengthens the divisibility conditions.
First
any rational root fully reduced would have to have a numerator that divides evenly into 1 and a denominator that divides evenly into 2. Hence the only possible rational roots are ±1/2 and ±1; since neither of these equates the polynomial to zero, it has no rational roots.
Second
the only possible rational roots would have a numerator that divides 6 and a denominator that divides 1, limiting the possibilities to ±1, ±2, ±3, and ±6. Of these, 1, 2, and –3 equate the polynomial to zero, and hence are its rational roots.
What is the rational root test?
The Rational Roots Test (also known as Rational Zeros Theorem) allows us to find all possible rational roots of a polynomial. Suppose a is root of the polynomial Pleft ( x right) that means Pleft ( a right) = 0. In other words, if we substitute a into the polynomial Pleft ( x right) and get zero, 0, it means that the input value is a root of the function.
What does it mean when a polynomial gets zero?
If you plug in each value to the given polynomial and gets zero, that means the number you substituted is a root! Try this on paper, and you should be convinced that there are only three values satisfying this condition.
What is the Rational Root Theorem?
The Rational Root Theorem is used in math to find the possible rational roots of a polynomial function, most specifically when the function is not factorable. These rational roots can also be called: x-intercepts, zeros or solutions. To solve for them set the polynomial equal to 0, as y=0 on our x-axis.
Rational Root Theorem Examples
Here are a few examples to show how the Rational Root Theorem is used.
Example 1: Finding Rational Roots
Using the polynomial {eq}f (x) = x^3 + x^2 + x - 3 {/eq} answer the following questions.
What does rational mean in English?
English Language Learners Definition of rational. : based on facts or reason and not on emotions or feelings. : having the ability to reason or think about things clearly. See the full definition for rational in the English Language Learners Dictionary.
What does rational mean in medical terms?
Medical Definition of rational. 1 a : having reason or understanding. b : relating to, based on, or agreeable to reason a rational explanation rational behavior. 2 : using medical treatments based on reason or general principles —used especially of an ancient school of physicians — compare empirical sense 1a.
What does "I'm sure there is a rational decision" mean?
1 : based on facts or reason and not on emotions or feelings a rational decision/choice I'm sure there is a rational [=sensible, reasonable] explanation for his decision.